The over-reinforced section is considered critical when compared with the under-reinforced condition. If over-reinforced, the section can fail suddenly without warning as a result of crushing the concrete.
However, in under-reinforced conditions, the section provides more warning prior to the failure.
Therefore, the designer should have a good understanding of the there conditions namely balance, under reinforced, and under reinforced conditions.
The article Balance, Over Reinforced and Under Reinforced could be referred to for more information on each type and failure mode.
It should be noted that over reinforced section does not fail suddenly. It will fail when the applied moment exceeds the moment capacity. When the section fails, there will not be warnings prior to the failure like in under reinforced sections.
The evaluation of the section is done as per the BS Standards (BS 8110).
The following will be checked in this worked example.
- Wheather section is over-reinforced?
- Bending moment capacity of the over-reinforced section?
Let’s check with an example.
Consider the following section.
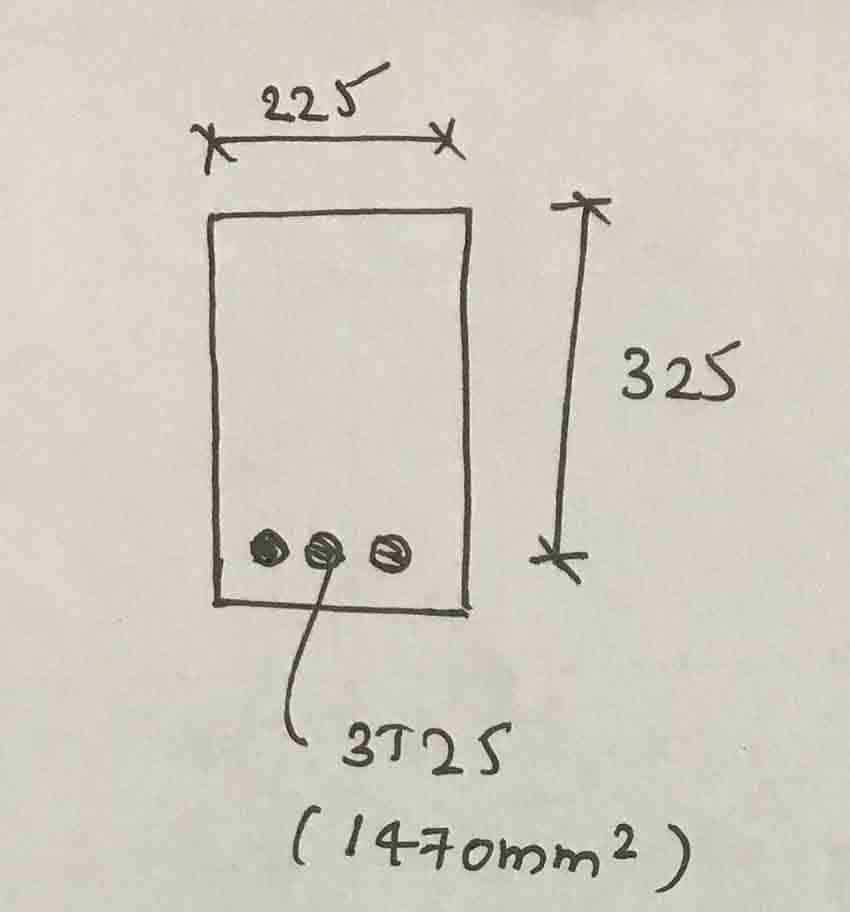
- Single reinforced section
- fcu = 25 N/mm2
- fy = 460 N/mm2
In this calculation, we consider the basic stress-strain relationship of the concrete section.
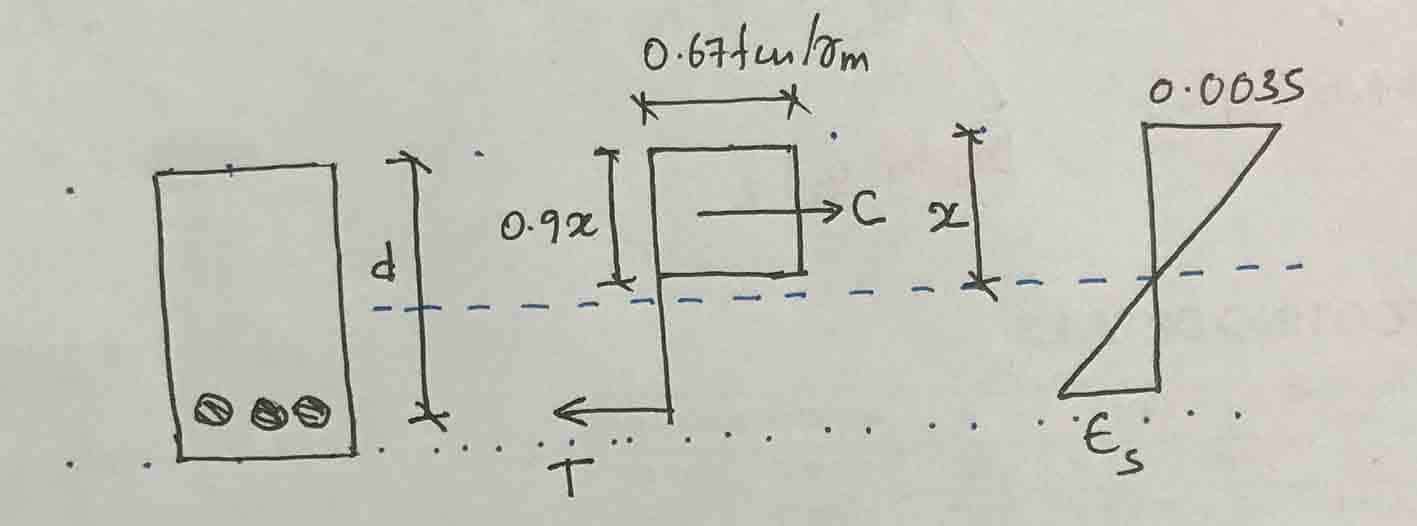
Firstly assume the reinforcement is yield.
T = 0.87fyAs = 0.87 x 460 x 1470 = 588,294 N
C = (0.67fcu/ϒm)(0.9x)b = (0.67×25/1.5)(0.9x)x225 = 226,125x
C = T
226,125 x = 588,294
x = 260 mm
x /d = 260 / 325 = 0.8 > 0.64
Section is over reinforced.
The assumption, steel is yield, is not not correct. We need to find the actual “x” by trial and error method. It need to be done until C = T.
Assume x = 225 mm
C = 226125 x = 226125 x 225 = 508781.25 N
Now calculate the steel strain to fined the steel stress. The above figure can be used to calculate the steel strain.
εs = 0.0035(325-225)/225 = 0.0016
Now let’s see the stress strain diagram.
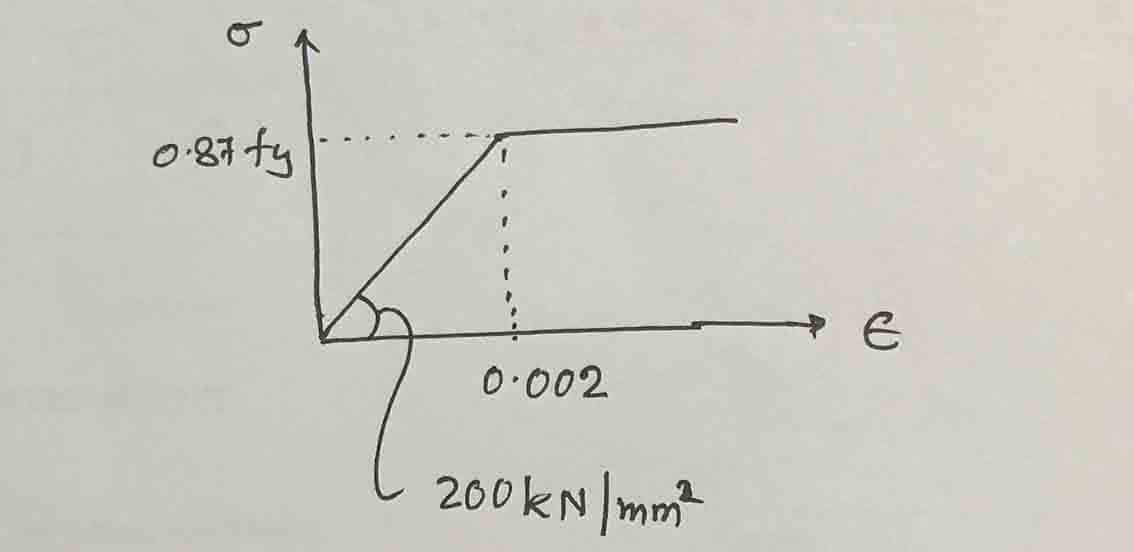
Steel stress, fs
fs = εs Es = 0.0016 x 200×103 = 320 N/mm2
T = 320 x 1470 = 470400 N
C ≠ T
We have to do several trials until C = T by assuming different neutral axis depths (x).
There is a different method to find the neutral axis depth to find directly. Since the steel is not yielded stress is less than the yield point (fs < 400 N/mm2 = 0.87×460).
Calculate the steel strain, εs
εs / 0.0035 = (d – x) / x
εs = 0.0035 (325 – x )/x
Now calculate steel stress
fs = εs Es = [0.0035 (325 – x )/x] 200×103
T = fs As = [0.0035 (325 – x )/x] 200×103 x 1470
C = (0.67fcu/ϒm)(0.9x)b = (0.67×25/1.5)(0.9x)x225 = 2278.125x
T = C
[0.0035 (325 – x )/x] 200×103 x 1470 = 2278.125x
x2 + 451.69x – 146798.35 = 0
x = 218.91 or -679.6; x should be positive
Therefore, x = 218.91 mm
Now calculate the bending capacity of the seciton
C = 2278.125 x 218.91 = 498704.34
lever arm
Z = d – 0.45x = 325 – 0.45 x 218.91 = 226.49 mm
M = Cz = 498704.34 x 226.49 / 10-6 = 112.95 kNm
The bending moment capacity of the over-reinforced section is 112.95 kNm.
You may refer to the Wikipedia article Reinforcement concrete for information on them.