Stability Checks for Retaining Walls
Overturning and sliding checks are done to make sure the stability of retaining walls. In addition to this, base bearing pressure is also checked to confirm whether it is within the limit. In this calculation, we are concentrating on the two stability checks of sliding and overturning.
Assumptions
- Friction angle of soil 30
- Friction coefficient between soil and concrete 0.5
- Dry density of soil 18 kN/m3
- Water table is not considered for this calculation(If the water pressure is considered, we have to consider the submerge unit weight of the soil and also need to consider the saturated unit weight of the soil depending on the capillary action of the soil.
- No surcharge load is considered to simplify the calculation
Active soil pressure = (1 – Sinϕ) / (1 + Sinϕ)
= (1 – Sin30) / (1 + Sin30)
= 0.333
Passive soil pressure = (1 + Sinϕ) / (1 – Sinϕ)
= (1+ Sin30) / (1 – Sin30)
= 3.0
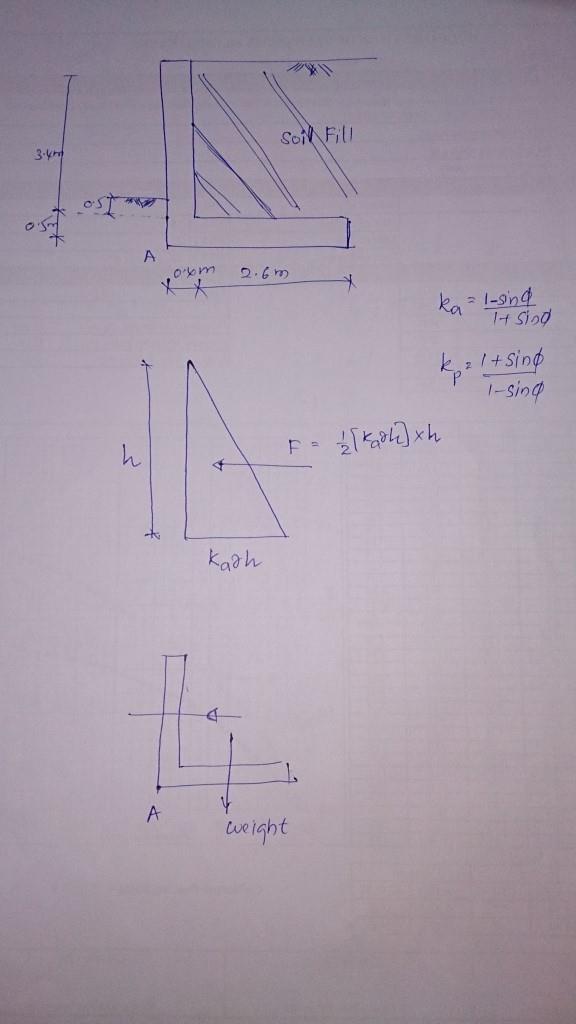
Check for Overturning
Pressure at base = Ka γ h
= 0.333x18x3.9
= 23.377 kN/m2
Lateral Force = 0.5×23.377×3.9
= 45.584 kN
Soil weigh on foundation = 2.6×3.4×18
= 159.12 kN
If the retaining wall is overturned, it will rotate around the point “A”. Therefore take moment around point ‘A’ to find the overturning moment and restoring moment.
Overturning Moment = 45.584 x 3.9 / 3
= 59.259 kNm
Restoring Moment = 159.12 x (2.6/2 +0.4)
= 270.504 kNm
Actual Factor of Safety = 270.504 / 59.259
= 4.565
It should be noted that the weight of the structure has not been considered in this calculation. Even without the weight of the structure, there is a higher restoring moment.
The factor of safety against sliding is generally considered as 1.5. However, it could be vary depending on the design requirements. Here, the value is well above the required value. Therefore, it can conclude as an overturning check is satisfactory.
Check of Sliding
Sliding can be considered in two ways. The friction force between soil and concrete or passive pressure generated by a shear key can be considered to avoid the sliding of the structure.
Case 01: No shear key, consider only friction between soil and concrete
The weight of the structure should be considered for this calculation as it increases the reaction that leads to a higher friction force.
Density of concrete = 24 kN/m3
Weight of the structure = (3.9×0.4 + 2.6×0.5)x24
= 68.64 kN
Total weight = weight of soil + weight of the structure
= 159.12 + 68.64
= 227.76 kN
Assume friction coefficient between soil and concrete as 0.5. This value may be vary depending on the ground condition.
Friction force = 0.5 x 227.76
= 113.88 kN
Lateral force = 45.584 kN
Factor of safety = 113.88 / 45.584
= 2.5
Generally, we keep the factor of safety around 1.5 against sliding. The actual safety factor is well above the allowable value. Therefore sliding is ok.
Case 02: Providing Shear Key
Some of the engineers are reluctant to use the friction force along to avoid the sliding of retaining walls as the ground conditions could be varied and unpredictable. In addition, when the height of the retaining wall is increasing, the effect of the passive pressure is considered most of the time. Even though the above calculation is satisfactory for sliding, we could proceed with this method also to get an idea about the concept.
Height of the shear key can be determined as follows
Factor of safety = Allowable sliding force / Actual sliding force
Allowable sliding force = FOS (actual sliding force)
0.5 (Kp γ h) h = 1.5 x 45.584
h2 = 1.5 x 45.584 / (0.5 Kp γ)
= 1.5 x 45.584 / (0.5x3x18)
= 2.532
h = 1.6 m
1.6 m height soil layer is required to generate above passive pressure if we assume fully mobilization of passive pressure and neglect the friction under the foundation.
As per the above figure, soil cover above the base is 0.5 m and the thickness of the base is 0.5m.
Depth of the shear key = 1.6 – 0.5 – 0.5
= 0.6 m
Provide a shear key of height 600mm.
It also notes that we need to check the pressure under the foundation and it should bellow the allowable bearing capacity of the soil.


