WATER LOAD DUE TO AN EARTHQUAKE – An Overview
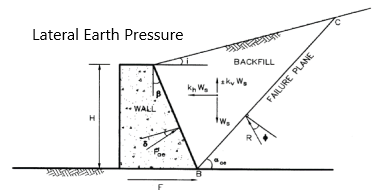
Hydrodynamic force due to the water above the ground level on the water retaining structures is discussed as per the guidelines are given in the EM 1110-2-2502 1989. However, there could be more recently published standard that could serve the same purpose.
The derivations are based on the inertia force distribution. The following figure indicates the variation of the water pressure with the height. The figure was extracted from EM 1110-2-2502.
The total hydrodynamic force can be calculated from the following equation which is described as Westergaadrd’s (1933) equation.
PE = (2/3) CE kh h2
Where,
PE – Total hydrodynamic force
CE – Factor depending on the depth of water
Kh – Horizontal earthquake coefficient
h – Water height
CE is a factor depending on the depth of water (h) and the earthquake period of vibration (T). In this equation, h shall be in feet and T shall be in seconds.
CE = 0.051 / [1-0.72 (h/1000T)2]0.5
Normally, for retaining and floodwalls, CE can be taken as 0.051. Further, the variation of the pressure at any point can be calculated from the following equation.
PE = CE kh (hy)0.5
However, as per the EM 1110-2-2100: 2005 which is published more recently, indicate different formula for evaluating the hydrodynamic water pressure and It is a modification to the formula given in EM 1110-2-2502: 1989. Thus, the following formula could be used to evaluate the water pressure load due to the earthquake.
of the pressure at any point can be calculated from the following equation.
PE = CE kh (hy)0.5
However, as per the EM 1110-2-2100: 2005 which is published more recently, indicate different formula for evaluating the hydrodynamic water pressure and It is a modification to the formula given in EM 1110-2-2502: 1989. Thus, the following formula could be used to evaluate the water pressure load due to the earthquake.
PE = (7/12) khγwh2
Total water pressure shall be calculated adding the static water pressure and the dynamic water pressure.